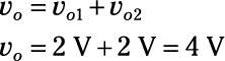Use superposition to analyze circuits that have lots of voltage and current sources. Superposition helps you to break down complex linear circuits composed of multiple independent sources into simpler circuits that have just one independent source. The total output, then, is the algebraic sum of individual outputs from each independent source.
Analyze circuits with two voltage sources
With the help of superposition, you can break down the complex circuit shown here into two simpler circuits that have just one voltage source each. To turn off a voltage source, you replace it with a short circuit.
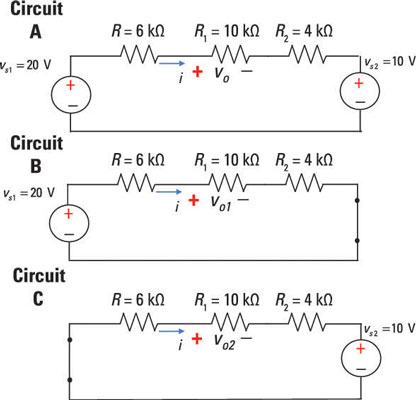
Circuit A contains two voltage sources, vs1 and vs2, and you want to find the output voltage vo across the 10-kΩ resistor. The next diagram shows the same circuit with one voltage source turned off: Circuit B contains one voltage source, with vs2 turned off and replaced by a short circuit. The output voltage due to vs1 is vo1.
Similarly, Circuit C is Circuit A with the other voltage source turned off. Circuit C contains one voltage source, with vs1 replaced by a short circuit. The output voltage due to voltage source vs2 is vo2.
Summing up the two outputs due to each voltage source, you wind up with the following output voltage:
To find the output voltages for Circuits B and C, you use voltage divider techniques. That is, you use the idea that a circuit with a voltage source connected in series with resistors divides its source voltage proportionally according to the ratio of a resistor value to the total resistance.
In Circuit B, you simply find the output voltage vo1 due to vs1 with a voltage divider equation:
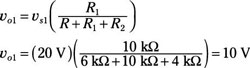
In Circuit C, finding the output voltage vo2 due to vs2 also requires a voltage divider equation, with the polarities of vo2 opposite vs2. Using the voltage divider method produces the output voltage vo2 as follows:
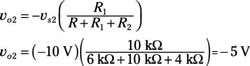
Adding up the individual outputs due to each source, you wind up with the following total output for the voltage across the 10-kΩ resistor:
When the sources are two current sources
The plan in this section is to reduce the circuit shown here to two simpler circuits, each one having a single current source, and add the outputs using superposition.
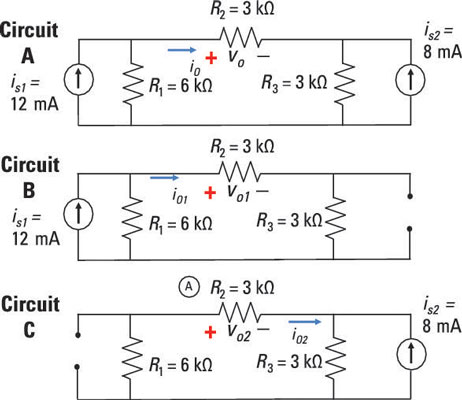
You consider the outputs from the current sources one at a time, turning off a current source by replacing it with an open circuit.
Circuit A consists of two current sources, is1 and is2, and you want to find the output current io flowing through resistor R2. Circuit B is the same circuit with one current source turned off: Circuit B contains one current source, with is2 replaced by an open circuit. The output voltage due to is1 is io1.
Similarly, Circuit C is Circuit A with only current source, with is1 replaced by an open circuit. The output current due to current source is2 is io2.
Adding up the two current outputs due to each source, you wind up with the following net output current through R2:
To find the output currents for Circuits B and C, you use current divider techniques. That is, you use the idea that for a parallel circuit, the current source connected in parallel with resistors divides its supplied current proportionally according to the ratio of the value of the conductance to the total conductance.
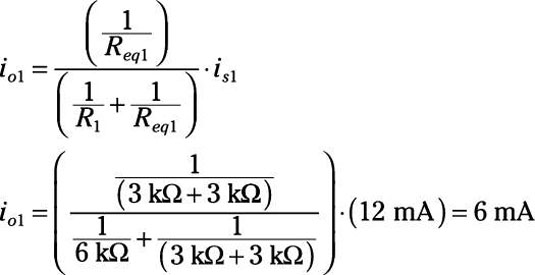
For Circuit B, you find the output current io1 due to is1 using a current divider equation. Note that there are two 3-kΩ resistors connected in series in one branch of the circuit, so use their combined resistance in the equation. Given Req1 = 3 kΩ + 3 kΩ and R1 = 6 kΩ, here’s output current for the first current source:
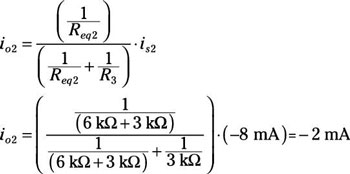
In Circuit C, the output current io2 due to is2 also requires a current divider equation. Note the current direction between io2 and is2: is2is opposite in sign to io2. Given Req2 = 6 kΩ + 3 kΩ and R3 = 3 kΩ, the output current from the second current source is
Adding up io1 and io2, you wind up with the following total output current:
When there is one voltage source and one current source
You can use superposition when a circuit has a mixture of two independent sources, with one voltage source and one current source. You need to turn off the independent sources one at a time. To do so, replace the current source with an open circuit and the voltage source with a short circuit.
Circuit A of the sample circuit shown here has an independent voltage source and an independent current source. How do you find the output voltage vo as the voltage across resistor R2?
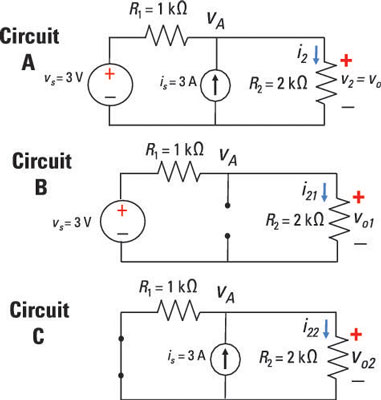
Circuit A (with its two independent sources) breaks up into two simpler circuits, B and C, which have just one source each. Circuit B has one voltage source because the current source was replaced with an open circuit. Circuit C has one current source because the voltage source was replaced with a short circuit.
For Circuit B, you can use the voltage divider technique because its resistors, R1 and R2, are connected in series with a voltage source. So here’s the voltage vo1 across resistor R2:
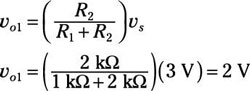
For Circuit C, you can use a current divider technique because the resistors are connected in parallel with a current source. The current source provides the following current i22 flowing through resistor R2:
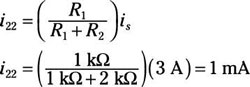
You can use Ohm’s law to find the voltage output vo2 across resistor R2:
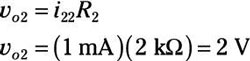
Now find the total output voltage across R2for the two independent sources in Circuit C by adding vo1 (due to the source voltage vs) and vo2 (due to the source current is). You wind up with the following output voltage: