One of the earliest applications of trigonometry was in measuring distances that you couldn't reach, such as distances to planets or the moon or to places on the other side of the world. Consider the following example.
The diameter of the moon is about 2,160 miles. When the moon is full, a person sighting the moon from the earth measures an angle of 0.56 degrees from one side of the moon to the other.
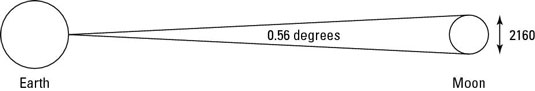
To figure out how far away the moon is from the earth, consider a circle with the earth at the center and the circumference running right through the center of the moon, along one of the moon's diameters. The moon is so far away that the straight diameter and slight curve of this big circle's circumference are essentially the same measure.
The arc that runs through the moon's diameter has an angle of 0.56 degrees and an arc length of 2,160 miles (the diameter). Using the arc-length formula, solve for the radius of the large circle, because the radius is the distance to the moon. To solve for the radius:
First, change 0.56 degrees to radians.


Input the numbers into the arc-length formula,

Enter 0.00977 radians for the radian measure and 2,160 for the arc length: 2,160 = 0.00977 x r.
Divide each side by 0.00977.
The distance to the moon is





