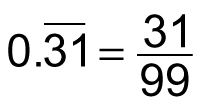To convert a fraction to a decimal, divide the numerator (top number) by the denominator (bottom number), using either calculator or pencil and paper. For example, here’s how to convert the fraction
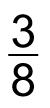
to a decimal:
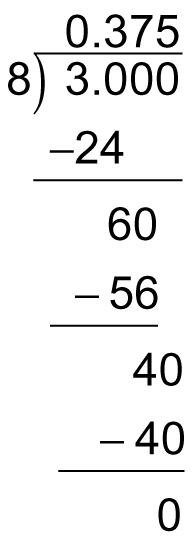
So,
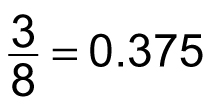
To convert a decimal to a fraction, begin by placing that decimal over the number 1. Then keep multiplying both the numerator (top number) and denominator (bottom number) by 10 until both are whole numbers. For example, here’s how to convert the decimal 0.13 to a fraction:
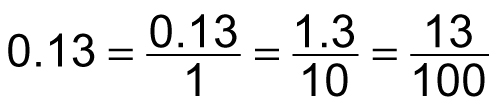
In both of these cases, the decimal forms of these numbers are terminating decimals — that is, the decimal can be written exactly in a finite number of decimal places.
In other cases, however, a decimal is repeating — that is, it cannot be written exactly without the numbers repeating forever.
Converting fractions to repeating decimals
Every fraction can be written as a decimal, either terminating or repeating. To write a fraction as a decimal, divide the numerator by the denominator. For example, here’s how you convert the fraction
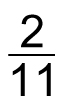
to a decimal:
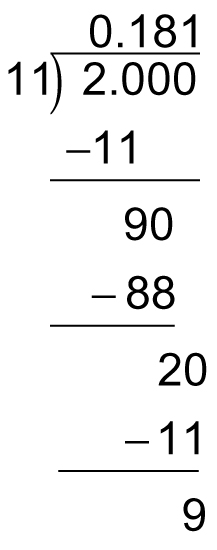
You can see that this decimal will never end, but instead will repeat forever in a pattern of 1s and 8s. Therefore,
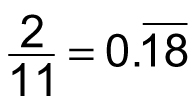
The bar over the numbers 18 means that these numbers are repeated infinitely: 0.1818181818. . . .
As another example, convert the fraction

to a decimal by dividing:

As in the previous example, the pattern of numbers in the answer repeats itself and will do so indefinitely. So,
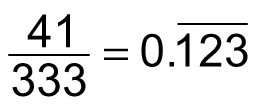
Converting repeating decimals to fractions
Every repeating decimal can be written as a fraction. A quick trick for converting a repeating decimal is to place the repeating numbers in the numerator of a fraction over the same number of 9s, and then reduce if necessary. For example, here’s how you convert the repeating decimals
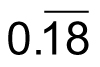
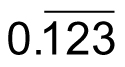
and
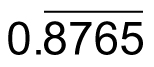
to fractions:
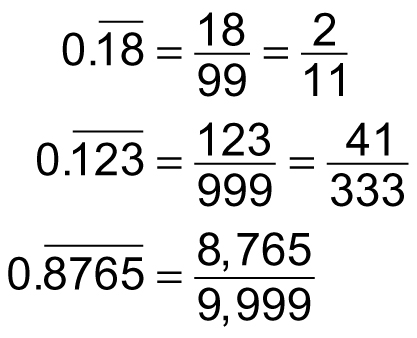
To gain insight into why this trick works, here is a step-by-step way to convert a repeating decimal to a fraction using algebra. Suppose you want to convert the decimal
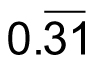
to a fraction. Begin by letting x equal
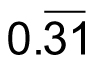
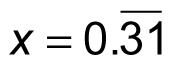
This decimal has two repeating decimal places, so multiply both sides of this equation by 100 — that is, the number that brings the whole repeating pattern to the left side of the decimal point:
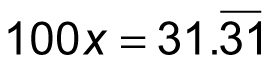
Note that this decimal still repeats forever. Now, subtract the original equation from this one:
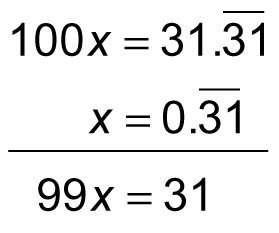
This step may seem strange, because on the right side of the equation you’re subtracting an infinite decimal from an infinite decimal. But this process removes the repeating decimal from the equation. Now, to solve for x, just divide by 99:
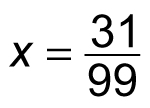
As you can see, the result shows that