How to Integrate Even Powers of Sines and Cosines

You can integrate even powers of sines and cosines. For example, if you wanted to integrate sin²x and cos²x, you would use these two half-angle trigonometry identities:

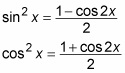
Here’s how you integrate cos²x:

1. Use the half-angle identity for cosine to rewrite the integral in terms of cos 2x:

   

2. Use the Constant Multiple Rule to move the denominator outside the integral:

   

3. Distribute the function and use the Sum Rule to split it into several integrals:

   

4. Evaluate the two integrals separately:

   

As a second example, here’s how you integrate sin² x cos⁴ x:

1. Use the two half-angle identities to rewrite the integral in terms of cos 2x:

   

2. Use the Constant Multiple Rule to move the denominators outside the integral:

   

3. Distribute the function and use the Sum Rule to split it into several integrals:

   

4. Evaluate the resulting odd-powered integrals:

   

With the integration behind you, use algebra to simplify the result. To start, combine the first and third terms, and second and fifth terms:

Now distribute the result: