Spherical coordinates are used — with slight variation — to measure latitude, longitude, and altitude on the most important sphere of them all, the planet Earth. Every point in space is assigned a set of spherical coordinates of the form
In case you’re not in a sorority or fraternity,
is the lowercase Greek letter rho,
is the lowercase Greek letter theta (commonly used in math to represent an angle),
is the lowercase Greek letter phi,
which is commonly pronounced either “fee” or “fye” (but never “foe” or “fum”).
The coordinate
corresponds to altitude. On the Earth, altitude is measured as the distance above or below sea level. In spherical coordinates, however, altitude indicates how far in space a point is from the origin.
The coordinate
corresponds to longitude: a measurement of angular distance from the horizontal axis.
The coordinate
corresponds to latitude. On the Earth, latitude is measured as angular distance from the equator. In spherical coordinates, however, latitude is measured as the angular distance from the North Pole.
Plotting
can be tricky at first. To get a feel for it, picture a globe and imagine traveling up and down along a single longitude line. Notice that as you travel, your latitude keeps changing, so
At the North Pole,

At the equator,

At the South Pole,

Some textbooks substitute the Greek letter
(rho) for r. Either way, the coordinate means the same thing: altitude, which is the distance of a point from the origin. In other textbooks, the order of the last two coordinates is changed around. Make sure you know which convention your book uses.
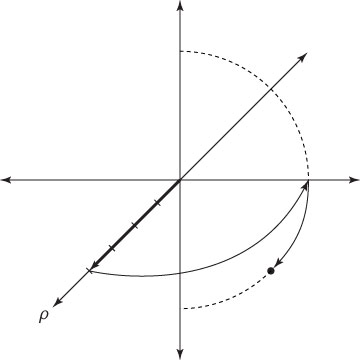
This figure shows you how to plot a point in spherical coordinates, in this case, the point
Follow these steps to plot this point:
Count 4 units outward in the positive direction from the origin on the horizontal axis.
Travel counterclockwise along the arc of a circle until you reach the line drawn at a

from the horizontal axis (again, as with polar coordinates).
Imagine a single longitude line arcing from the north pole of a sphere through the point on the equator where you are right now and onward to the south pole.
Travel down to the line of latitude at an angular distance of

from the north pole — that is, halfway between the equator and the south pole — and plot your point there.






