Getting Started with Trig Identities

You need to become more familiar with the possibilities for rewriting trigonometric expressions. A trig identity is really an equivalent expression or form of a function that you can use in place of the original. The equivalent format may make factoring easier, solving an application possible, and (later) performing an operation in calculus more manageable.

The trigonometric identities are divided into many different classifications. These groupings help you remember the identities and make determining which identity to use in a particular substitution easier.

In a classic trig identity problem, you try to make one side of the equation match the other side. The best way to do so is to work on just one side — the left or the right — but sometimes you need to work on both sides to see just how to work the problem to the end.

In pre-calculus, you’ll work with the basic trigonometric identities in the following ways:

• Determining which trig functions are reciprocals of one another

• Creating Pythagorean identities from a right triangle whose hypotenuse measures 1 unit

• Determining the sign of identities whose angle measure is negated

• Matching up trig functions and their co-functions

• Using the periods of functions in identities

• Making the most of selected substitutions into identities

• Working on only one side of the identity

• Figuring out where to go with an identity by working both sides at once

Don’t let common mistakes trip you up; keep in mind that when working on trigonometric identities, some challenges will include the following:

• Keeping track of where the 1 goes in the Pythagorean identities

• Remembering the middle term when squaring binomials involving trig functions

• Correctly rewriting Pythagorean identities when solving for a squared term

• Recognizing the exponent notation

Practice problems

1. Prove the trig identity. Indicate your first identity substitution:

   

   Answer: use the reciprocal identity

   Because each term contains a function and its reciprocal, using reciprocal identities will simplify the terms quickly.

   First, replace

   

   with its reciprocal identity,

   

   and

   

   with its reciprocal identity:

   

   Then simplify the complex fractions.

   

   Finally, replace

   

   with 1, using the Pythagorean identity: 1 = 1

2. Determine the missing term or factor in the identity by changing all functions to those using sine or cosine:

   

   Answer: 1

   Use the reciprocal identity to replace csc²x and use the ratio identity to replace tan²x:

   

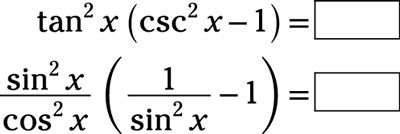
   Distribute

   

   simplify, and then combine the two terms:

   

   Rewrite the Pythagorean identity, sin²x + cos²x = 1, by subtracting sin²x from each side to get cos²x = 1 – sin²x. Replace the numerator of the fraction in the identity with cos²x:

   

   The missing term is 1.