A function consists of a rule that you apply to the input values. The result is a single output value. You can usually use a huge number of input values, and they're all part of the domain of the function. The output values make up the range of the function.
Domain of a trig function
The domain of a function consists of all the values that you can use as input into the function rule. The domain is another of that function's characteristics, because different functions have different numbers that you can input and have the outputs make any sense.
is a function whose domain can't contain any negative numbers, because the square root of a negative number isn't a real number.
The following function has a domain that can't include the number –3:
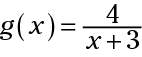
Any other real number is okay, but not –3, because putting a –3 in for x makes the denominator equal to 0, and you can't divide by 0. (A fraction with a 0 in the denominator represents a number that doesn't exist.) With trig functions, the domain (input values) is angle measures — either in degrees or radians.
Some of the trig functions have restrictions on their domains, too. For example, the tangent function has a domain that can't include 90 degrees or 270 degrees, among the many other restricted values.
Range of a trig function
The range of a function consists of all its output values — the numbers you get when you input numbers from the domain into the function and perform the function operations on them. Sometimes, a range can be all possible real numbers — it has no limit.
That situation happens in a function such as h(x) = 3x + 2. In this equation, both the domain and the range are unlimited. You can put in any real number, and you can get an output of any real number that you can possibly think of. Ranges can end up being restricted, though.
For example, the function k(x) = x2 + 6 will always have results that are either the number 6 or some positive number greater than 6. You can never get a negative number or a number less than 6 as an output. The ranges of some trig functions are restricted, too. For example, the output of the sine function never exceeds 1 or goes lower than –1.





