Fractions are your friends. You may not believe this now, but the more you work with trigonometry functions, the more you’ll like fractions. Finding a common denominator to combine fractions often paves the way to solving an identity.
In the identity
![]()
the two denominators on the left have nothing in common, so you multiply each fraction by the other’s denominator — or, rather, by that denominator over itself, which equals 1.
-
Multiply each fraction on the left by an equivalent of 1 to create a common denominator.

-
Multiply the fractions together and simplify the numerators.
Leave the denominator alone.

-
Replace the first numerator with its equivalent by using the Pythagorean identity.
The fractions are opposites of one another.

Now, prove the identity
![]()
by finding a common denominator.
-
Multiply each fraction on the left by the equivalent of 1, creating a common denominator.
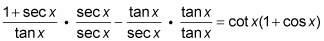
-
Simplify the numerators by multiplying out the fractions.

-
Replace tan2x with its equivalent by using the Pythagorean identity.
Then combine the two numerators.

-
Simplify the numerator.
Then rewrite the left side as the product of two fractions. You have to carefully select where to put the numerator — to your best advantage.

-
Multiply the fraction with sec x in the denominator by cos x divided by cos x, which is equivalent to 1.

-
Multiply out the second fraction, distributing through the numerator.

Because cos x and sec x are reciprocals, their product is 1.
-
Substitute 1 in both the numerator and the denominator.

-
Replace the reciprocal of tan x with cot x.






