A 30-60-90 right triangle has angles measuring just what the name says. The two acute, complementary angles are 30 and 60 degrees. These triangles are great to work with, because the angle measures, all being multiples of 30, have a pattern, and so do the measures of the sides.
Oh, yes, the Pythagorean theorem still holds — you have that relationship between the squares of the sides. But a, b, and c are related in another way, too. In a 30-60-90 right triangle, if a is the shortest side, then the hypotenuse, the longest side, measures twice that, or 2a.
You can use 2a instead of the letter c. And the middle length is
or about 1.7 times as long as the shortest side; this number replaces the letter b. The particularly nice part about this triangle is that you can write all three sides in terms of one variable, a. Look at how these lengths fit into the Pythagorean theorem:
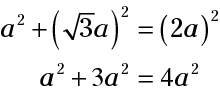
Here’s a sample problem you can solve by taking advantage of the special relationships within a 30-60-90 right triangle: If the hypotenuse of a 30-60-90 right triangle is 8 units long, then how long are the other two sides?
Find the length of the shorter leg.
The hypotenuse is twice as long as the shorter leg, a. So 8 = 2a. Divide by 2, and you get a = 4.
Find the length of the longer leg.
The longer leg is





