If you know the radius of a circular track, you can use physics to calculate how fast an object needs to move in order to stay in contact with the track without falling when it reaches the top of the loop.
Maybe you’ve watched extreme sports on television and wondered how bikers or skateboarders can ride into a loop on a track and go upside down without falling to the ground. Shouldn’t gravity bring them down? How fast do they have to go? The answers to these vertical circular-motion questions lie in centripetal force and the force of gravity.
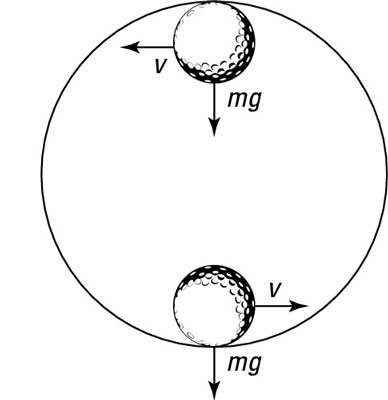
Take a look at the figure, where a ball is looping around a circular track. A question you may come across in introductory physics classes asks, “What speed is necessary so that the ball makes the loop safely?” The crucial point is at the very top of the track — if the ball is going to peel away from its circular track, the top is where it’ll fall. To answer the crucial question, you must know what criterion the ball must meet to hold on. Ask yourself, “What’s the constraint that the ball must meet?”
To travel in a loop, an object must have a net force acting on it that equals the centripetal force it needs to keep traveling in a circle of the given radius and speed. At the top of its path, as you can see in the figure, the ball barely stays in contact with the track. Other points along the track provide normal force because of the speed and the fact that the track is curved. If you want to find out what minimum speed an object needs to have to stay on a loop, you need to look at where the object is just barely in contact with the track — in other words, on the verge of falling out of its circular path.
The normal force the track applies to an object at the top is just about zero. The only force keeping the object on its circular track is the force of gravity, which means that at the apex, the speed of the object has to be such that the centripetal force equals the object’s weight to keep it going in a circle whose radius is the same as the radius of the loop. That means that if this is the force needed
then the force of gravity at the top of the loop is
Fg = mg
And because Fg must equal Fc, you can write
You can simplify this equation into the following form:
The mass of any object, such as a motorcycle or a race car, that is traveling around a circular track drops out of the equation.
The square root of r times g is the minimum speed an object needs at the top of the loop in order to keep going in a circle. Any slower object will peel off the track at the top of the loop (it may drop back into the loop, but it won’t be following the circular track at that point). For a practical example, if the loop from the figure has a radius of 20.0 meters, how fast does the ball have to travel at the top of the loop in order to stay in contact with the track? Just put in the numbers:
At the top of the track, the golf ball has to travel 14.0 meters per second, which is about 31 miles per hour.





