Calculating the Force Needed to Move an Object Up a Slope

In physics, when frictional forces are acting on a sloped surface such as a ramp, the angle of the ramp tilts the normal force at an angle. When you work out the frictional forces, you need to take this fact into account.

Normal force, N, is the force that pushes up against an object, perpendicular to the surface the object is resting on. The normal force isn’t necessarily equal to the force due to gravity; it’s the force perpendicular to the surface an object is sliding on. In other words, the normal force is the force pushing the two surfaces together, and the stronger the normal force, the stronger the force due to friction.

You must battle gravity and friction to push an object up a ramp.

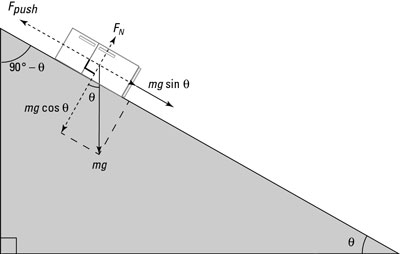
What if you have to push a heavy object up a ramp? Say, for example, you have to move a refrigerator. You want to go camping, and because you expect to catch plenty of fish, you decide to take your 100-kilogram refrigerator with you. The only catch is getting the refrigerator into your vehicle (see the figure). The refrigerator has to go up a 30-degree ramp that happens to have a static coefficient of friction with the refrigerator of 0.20 and a kinetic coefficient of friction of 0.15. The good news is that you have two friends to help you move the fridge. The bad news is that you can supply only 350 newtons of force each, so your friends panic.

The minimum force needed to push that refrigerator up the ramp has a magnitude F_push, and it has to counter the component of the weight of the refrigerator acting along the ramp and the force due to friction.

The first step in this problem is to resolve the weight of the refrigerator into components parallel and perpendicular to the ramp. Take a look at the figure, which shows the refrigerator and the forces acting on it. The component of the weight of the refrigerator along the ramp is

and the component of the refrigerator’s weight perpendicular to the ramp is

When you know the component of the weight along the ramp, you can work out the minimum force required to push the refrigerator up the ramp. The minimum force has to overcome the static force of friction acting down the ramp and the component of the refrigerator’s weight acting down the ramp, so the minimum force is

The next question is, “What’s the force of friction, F_F?” Should you use the static coefficient of friction or the kinetic coefficient of friction? Because the static coefficient of friction is greater than the kinetic coefficient of friction, the static coefficient is your best choice. After you and your friends get the refrigerator to start moving, you can keep it moving with less force. Because you’re going to use the static coefficient of friction, you can get F_F this way:

You also need the normal force, F_N, to continue. F_N is equal and opposite to the component of the refrigerator’s weight acting perpendicularly to the ramp. The component of the refrigerator’s weight acting perpendicularly to the ramp is

so you can say that the normal force acting on the refrigerator is

You can verify this by letting theta go to zero, which means that F_N becomes mg, as it should.

The static force of friction, F_F, is then given by

So the minimum force required to overcome the component of the weight acting along the ramp and the static force of friction is given by

Now just plug in the numbers:

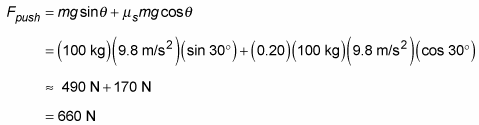
You need 660 newtons of force to push the refrigerator up the ramp. In other words, your two friends, who can exert 350 newtons each, are enough for the job.