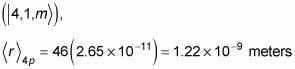Calculate the Distance of an Electron from the Proton of a Hydrogen Atom

When you want to find where an electron is at any given time in a hydrogen atom, what you’re actually doing is finding how far the electron is from the proton. You can find the expectation value of r, that is, , to tell you its location. Given that the wave function is

the following expression represents the probability that the electron will be found in the spatial element d³r:

In spherical coordinates,

So you can write

as

The probability that the electron is in a spherical shell of radius r to r + dr is therefore

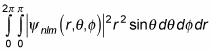
And because

this equation becomes the following:

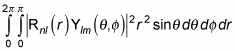
The preceding equation is equal to

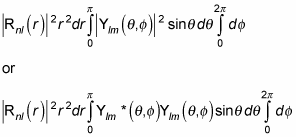
(Remember that the asterisk symbol [*] means the complex conjugate. A complex conjugate flips the sign connecting the real and imaginary parts of a complex number.)

Spherical harmonics are normalized, so this just becomes

Okay, that’s the probability that the electron is inside the spherical shell from r to r + dr. So the expectation value of r, which is , is

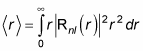
which is

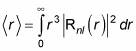
This is where things get more complex, because R_nl(r) involves the Laguerre polynomials. But after a lot of math, here’s what you get:

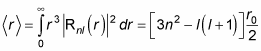
where r₀ is the Bohr radius:

The Bohr radius is about

so the expectation value of the electron’s distance from the proton is

So, for example, in the 1s state

the expectation value of r is equal to

And in the 4p state