Determining the Radial Part of a Wave Function

In quantum physics, you can determine the radial part of a wave function when you work on problems that have a central potential. With central potential problems, you’re able to separate the wave function into a radial part (which depends on the form of the potential) and an angular part, which is a spherical harmonic.

You can give the radial part of the wave function the name R_nl(r), where n is a quantum number corresponding to the quantum state of the radial part of the wave function and l is the total angular momentum quantum number. The radial part is symmetric with respect to angles, so it can’t depend on m, the quantum number of the z component of the angular momentum. In other words, the wave function for particles in central potentials looks like the following equation in spherical coordinates:

The next step is to solve for R_nl(r) in general. Substituting

from the preceding equation into the Schrödinger equation,

gives you

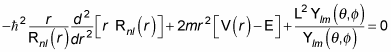
Okay, what can you make of this? First, note that the spherical harmonics are eigenfunctions of L² (that’s the whole reason for using them), with eigenvalue

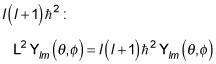
So the last term in this equation is simply

That means that

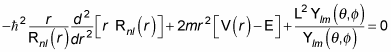
takes the form

which equals

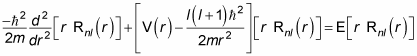
The preceding equation is the one you use to determine the radial part of the wave function, R_nl(r). It’s called the radial equation for a central potential.

When you solve the radial equation for R_nl(r), you can then find

because you already know

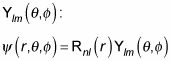
So, you’re simply finding the solution to the radial equation.