Solving the Wave Function of R Using the Schrödinger Equation

If your quantum physics instructor asks you to solve for the wave function of the center of mass of the electron/proton system in a hydrogen atom, you can do so using a modified Schrödinger equation:

What you will find is that you can actually ignore

and go straight on to

Here’s how it works.

Because the Schrödinger equation contains terms involving either R or r but not both, the form of this equation indicates that it’s a separable differential equation. And that means you can look for a solution of the following form:

Substituting the preceding equation into the one before it gives you the following:

And dividing this equation by

gives you

This equation has terms that depend on either

but not both. That means you can separate this equation into two equations, like this (where the total energy, E, equals E_R + E_r):

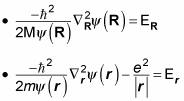
Multiplying

gives you

And multiplying

gives you

Now you have two Schrödinger equations, which you can solve independently.

So, using

you can now solve for

which is the wave function of the center of mass of the electron/proton system. This is a straightforward differential equation, and the solution is

Here, C is a constant and k is the wave vector, where

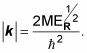
In practice, however, E_R is so small that people almost always just ignore

— that is, they assume it to be 1. In other words, the real action is in

is the wave function for the center of mass of the hydrogen atom, and

is the wave function for a (fictitious) particle of mass m.