In econometrics, a random variable with a normal distribution has a probability density function that is continuous, symmetrical, and bell-shaped. Although many random variables can have a bell-shaped distribution, the density function of a normal distribution is precisely
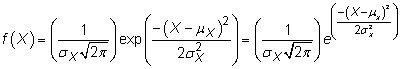
where
represents the mean of the normally distributed random variable X,
is the standard deviation,and
represents the variance of the normally distributed random variable.
A shorthand way of indicating that a random variable, X, has a normal distribution is to write
A distinctive feature of a normal distribution is the probability (or density) associated with specific segments of the distribution. The normal distribution in the figure is divided into the most common intervals (or segments): one, two, and three standard deviations from the mean.
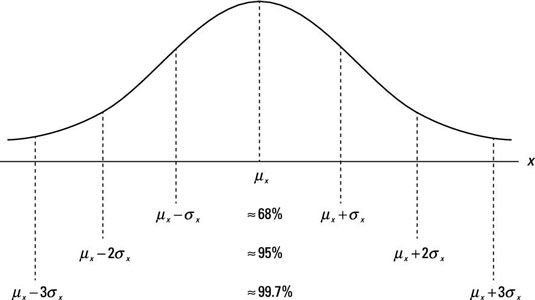
With a normally distributed random variable, approximately 68 percent of the measurements are within one standard deviation of the mean, 95 percent are within two standard deviations, and 99.7 percent are within three standard deviations.
Suppose you have data for the entire population of individuals living in retirement homes. You discover that the average age of these individuals is 70, the variance is 9
and the distribution of their age is normal. Using shorthand, you could simply write this information as
If you randomly select one person from this population, what are the chances that he or she is more than 76 years of age?
Using the density from a normal distribution, you know that approximately 95 percent of the measurements are between 64 and 76
(notice that 6 is equal to two standard deviations). The remaining 5 percent are individuals who are less than 64 years of age or more than 76. Because a normal distribution is symmetrical, you can conclude that you have about a 2.5 percent (5% / 2 = 2.5%) chance that you randomly select somebody who is more than 76 years of age.
If a random variable is a linear combination of another normally distributed random variable(s), it also has a normal distribution.
Suppose you have two random variables described by these terms:
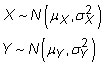
In other words, random variable X has a normal distribution with a mean of
and variance of
and random variable Y has a normal distribution with a mean of
and a variance of
If you create a new random variable, W, as the following linear combination of X and Y, W = aX + bY, then W also has a normal distribution. Additionally, using expected value and variance properties, you can describe the new random variable with this shorthand notation:






