Measures of central dispersion show how "spread out" the elements of a data set are from the mean. Three of the most commonly used measures of central dispersion include the following:
Range
Variance
Standard deviation
Range
The range of a data set is the difference between the largest value and the smallest value. You compute it the same way for both samples and populations.
Variance
You can think of the variance as the average squared difference between the elements of a data set and the mean. The formulas for computing a sample variance and a population variance are slightly different.
Here is the formula for computing sample variance:
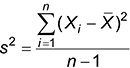
And here is the formula for computing population variance:
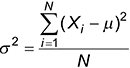
Standard deviation
The standard deviation is simply the square root of the variance. It's more commonly used as a measure of dispersion than the variance because it's measured in the same units as the elements of the data set, whereas the variance is measured in squared units.





