Coupons are a very effective way to price discriminate. Customers who are very responsive to price changes — that is, customers with a very elastic demand — are likely to take time to find coupons that effectively lower the good’s price. On the other hand, customers less responsive to price changes because of their less elastic demand aren’t as likely to take the time to find coupons.
When you use coupons, you start by establishing a single price for the good. The price is then lower for customers possessing a coupon. So, customers not using a coupon pay the price P, while customers using the coupon pay the price P – C, where C represents the coupon’s value.
In essence, coupons are another way to implement third-degree price discrimination. The rule for maximizing profit with third-degree price discrimination is
Marginal revenue is a function of the price elasticity of demand, or
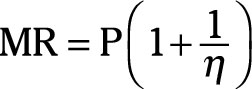
Assume that customers in group A have the less elastic demand; therefore, they pay the good’s full price P. Customers in group B have the more elastic demand and use a coupon. These customers essentially pay the price P – C for the good. Combining the last two equations with this information yields
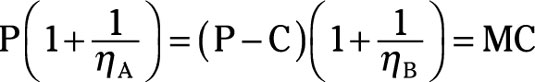
where P is the good’s price, C is the coupon’s value, çAis the price elasticity of demand for group A customers who have a less elastic demand, çB is the price elasticity of demand for group B customers who have a more elastic demand, and MC is marginal cost.
For example, you own a restaurant at a vacation destination. Your customers fall into two major groups — vacation travelers who must eat out and have a less elastic demand and local residents who can stay home and eat and thus have a more elastic demand for restaurant meals.
Assume that the price elasticity of demand for vacation travelers is –1.5 and the price elasticity of demand for local residents is –3. In addition, the marginal cost of providing a meal, including labor, ingredients, and so on, is $6.00.
Use the following steps to determine the price to charge for a meal and the coupon’s value:
The following equation maximizes profit.
In the equation, P is the price of a restaurant meal in dollars, C is the coupon’s value in dollars, çV is the price elasticity of demand for vacation travelers, çL is the price elasticity of demand for local residents, and MC is marginal cost in dollars.

Determine the price for a restaurant meal.
Use the vacation traveler portion of the equation in Step 1 and marginal cost.

Determine the coupon’s value.
Use the price determined in Step 2 and the local resident portion of the equation in Step 1.

Your restaurant should establish a price of $18.00 for a meal. It should provide $9.00 coupons to local residents. One method of providing coupons to local residents that would generally exclude vacation travelers is to use advertising mailers delivered to local addresses or publish coupons in local newspapers.





