The solution to a definite integral gives you the signed area of a region. In some cases, signed area is what you want, but in some problems you’re looking for unsigned area.
The signed area above the x-axis is positive, but the signed area below the x-axis is negative. In contrast, unsigned area is always positive. The concept of unsigned area is similar to the concept of absolute value. So if it’s helpful, think of unsigned area as the absolute value of a definite integral.
In problems where you’re asked to find the area of a shaded region on a graph, you’re looking for unsigned area. But if you’re unsure whether a question is asking you to find signed or unsigned area, ask the professor. This goes double if an exam question is unclear. Most professors will answer clarifying questions, so don’t be shy to ask.
Suppose that you’re asked to calculate the shaded unsigned area that’s shown in this figure.
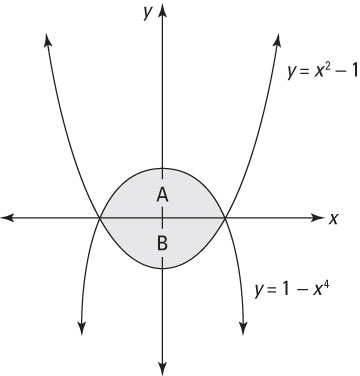
This area is actually the sum of region A, which is above the x-axis, and region B, which is below it. To solve the problem, you need to find the sum of the unsigned areas of these two regions.
Fortunately, both functions intersect each other and the x-axis at the same two values of x: x = –1 and x = 1. Set up definite integrals to find the area of each region as follows:
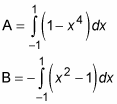
Notice that the definite integral for region B is negated to account for the fact that the definite integral produces negative area below the x-axis. Now just add the two equations together:
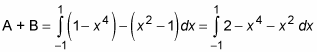
Solving this equation gives you the answer that you’re looking for (be careful with all those minus signs!):
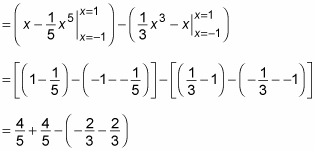
Notice at this point that the expression in the parentheses — representing the signed area of region B — is negative. But the minus sign outside the parentheses automatically flips the sign as intended:





