Certain mathematical expressions allow you to combine stretching, shrinking, translating, and reflecting a function all into one graph. An expression that shows all the transformations in one is
![]()
where
-
a is the vertical transformation.
-
c is the horizontal transformation.
-
h is the horizontal shift.
-
v is the vertical shift.
For instance, f(x) = –2(x – 1)2 + 4 moves the graph of y = x2 right 1 unit, vertically stretches it by a factor of 2, reflects it upside down, and then moves it up 4 units.

This figure shows each stage.
-
Figure a is the parent graph: k(x) = x2.
-
Figure b is the horizontal shift to the right by one: h(x) = (x – 1)2.
-
Figure c is the vertical stretch of two: f(x) = –2(x – 1)2. (Notice that because the value was negative, the graph was also turned upside down.)
-
Figure d is the vertical shift up by four: g(x) = –2(x – 1)2 + 4.
The following transformation illustrates the importance of the order of the process. You graph the function
![]()
with the following steps:
-
Rewrite the function in the form

Reorder the function so that the x comes first (in descending order). And don’t forget the negative sign! Here it is:

-
Factor out the coefficient in front of the x.
You now have

-
Reflect the parent graph.
Because the –1 is inside the square-root function, q(x) is a horizontal reflection over a vertical line of
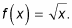
-
Shift the graph.
The factored form of q(x) (from Step 2) reveals that the horizontal shift is four to the right.

This figure shows the graph of
![]()






