A race car is going around a circular track. A photographer standing at the center of the track takes a picture, turns 80 degrees, and then takes another picture 10 seconds later. If the track has a diameter of 1/2 mile, how fast is the race car going? The figure shows the photographer in the middle and the car in the two different positions.
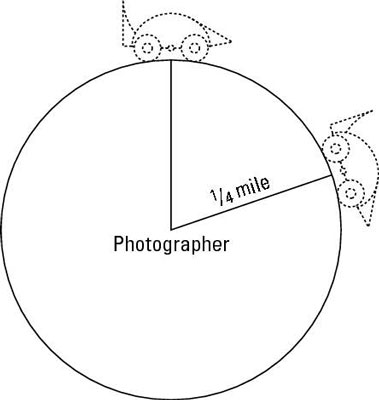
How fast is the car going? Where does the problem make any mention of speed? Actually, in this situation, the car travels partway around the track in 10 seconds. By computing the arc length, you can determine how fast the car is traveling.
A formula that you'll find mighty helpful is the one that says distance equals rate multiplied by time, where rate is miles per hour (or feet per second or some such measure), and time is the same measure as in the rate: d = r x t.
First, change the 80 degrees to radians.
You end up with

radians.
Input the numbers in the arc-length formula.
Putting in the radian measure and the radius of the track, 1/4 mile, you get

mile, which is the distance the car traveled in 10 seconds.
Multiply this result by 6 (since 10 seconds is 1/6 of a minute) to get miles traveled in a minute.
This calculation gives you 2.094 miles per minute.
Then multiply that number by 60 to get miles traveled in one hour.
This calculation gives you 125.64 miles. So the car is traveling about 126 mph.





