In physics, just as you can add two numbers to get a third number, you can add two vectors to get a resultant vector. To show that you’re adding two vectors, put the arrows together so that one arrow starts where the other arrow ends. The sum is a new arrow that starts at the base of the first arrow and ends at the head (pointy end) of the other.
Consider an example using displacement vectors. A displacement vector gives the change in position: the distance from the starting point to the ending point is the magnitude of the displacement vector, and the direction traveled is the direction of the displacement vector.
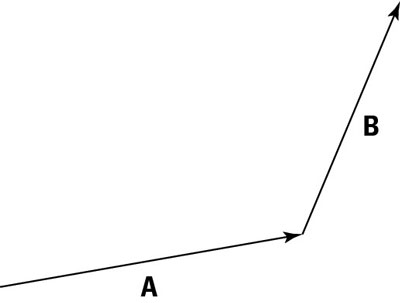
Assume, for example, that a passerby tells you that to get to your destination, you first have to follow vector A and then vector B. Just where is that destination? You work this problem just as you find the destination in everyday life. First, you drive to the end of vector A, and from that point, you drive to the end of vector B, just as you see in the above figure.
When you get to the end of vector B, how far are you from your starting point? To find out, you draw a vector, C, from your starting point (foot, or tail, of the first vector) to your ending point (head of the second vector), as you see in the below figure.
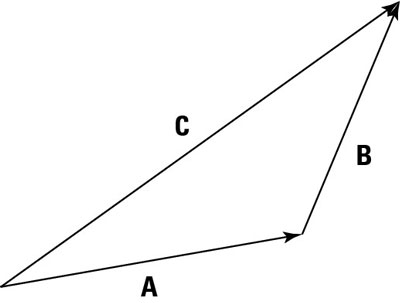
This new vector represents your complete trip, from start to finish. In other words, C = A + B. The vector C is called the sum, the result, or the resultant vector.






