In physics, when the pressure in a system changes but the volume is constant, you have what is called an isochoric process. An example of this would be a simple closed container, which can’t change its volume.
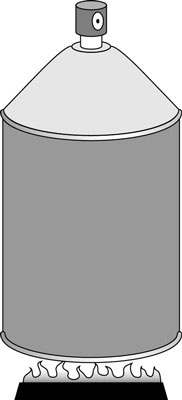
In the first figure, someone has neglectfully tossed a spray can onto a fire. As the gas inside the spray can heats up, its pressure increases, but its volume stays the same (unless, of course, the can explodes).
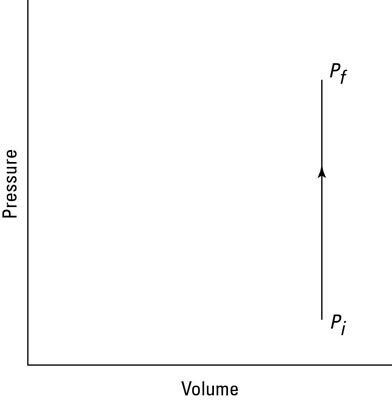
How much work does the fire do on the spray can? Look at the graph in the second figure. In this case, the volume is constant, so Fs (force times distance) equals zero. No work is being done — the area under the graph is zero.
Here’s an example. The CEO of Acme Pressure Vessels approaches you and says, “We’re adding 16,000 joules of energy to 5 moles of ideal gas at constant volume, and we want to know how much the internal energy changes. Can you help?”
You get out your clipboard and explain. The work done by an ideal gas depends on the change in its volume:
Because the volume change is zero in this case, the work done is zero.
The change in internal energy of an ideal gas is
Because W is zero, the following is true:
You turn to the CEO and say, “You’ve added 16,000 joules of energy to an ideal gas at constant volume, so the change in the gas’s internal energy is exactly 16,000 joules.”
“What?” says the CEO. “That was too easy. We won’t pay.”
Handing the CEO a receipt, you say, “You already have. Thanks for your business.”





