On the ACT Math test, you’ll probably have to multiply pairs of matrices that have either one row or one column. An easy way to multiply a horizontal matrix by a vertical matrix is to set up a small grid. This method allows you to fill in the numbers to get the right answer.
Matrix multiplication is not commutative, because the order in which you multiply two matrices can change the result. In other words, if P and Q are matrices, P multiplied by Q doesn’t necessarily equal Q multiplied by P.
Example
Here's an example of multiplying a horizontal matrix by a vertical matrix:
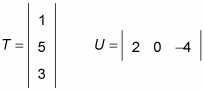
Which of the following is the result when you multiply U by T?
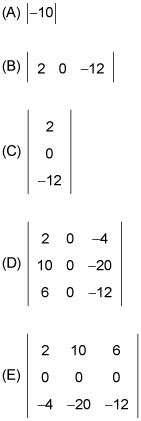
Notice that U has three columns and T has three rows, so you can multiply these two matrices in this order. To start, make a grid with U in the left-hand column and T in the top row:
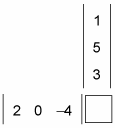
Notice that the resulting grid has only one box. To fill it in, multiply the three pairs of corresponding numbers in the two matrices, and then add the results:
Place this result into the box:
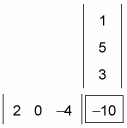
The contents of the grid become the result of the matrix multiplication:
So the correct answer is Choice (A).






